Some days ago, I was asked by a friend what my favourite number was. Since I have been intensively studying mathematics for a long time now (more intensive than most people anyway), I thought, Come on! That must be easy for someone like you!
It wasn't. My mind was completely blank.
Years ago, this question would have been incredibly easy for me; I guess that me studying mathematics rather made me lose my ability to connect numbers with emotions or other non-mathematical things. There is no special mathematical connection between any number and any emotion (at least none known today), and maybe my mind had to accept this fact in order to grasp the numbers' real essence. (I don't really know if I have yet reached this essence, but I am at the least a lot closer to it than I was before my mathematical studies.) Since mathematics opened before my eyes and mind the most beautiful world I have ever known (except music, maybe), I am not so very sad about me losing this ability.
When I was a child, I was a synesthete. 1 was black for me, 2 was yellow, three was red ... I still remember, but I have lost the ability itself. During my childhood, someone could have said "four", and I would immediately have seen a green four inside my head. It worked with letters. It worked with words.
At that time, I thought that was perfectly normal. I thought everybody saw these colors. I didn't know it is a special ability, nor did I know it is a medical term (not in the negative sense). If I had, I would have protected this gift. Now I have lost it. That I am sad about, because I could have done such wonderful things with it, especially since I have become an artist.
It wasn't. My mind was completely blank.
Years ago, this question would have been incredibly easy for me; I guess that me studying mathematics rather made me lose my ability to connect numbers with emotions or other non-mathematical things. There is no special mathematical connection between any number and any emotion (at least none known today), and maybe my mind had to accept this fact in order to grasp the numbers' real essence. (I don't really know if I have yet reached this essence, but I am at the least a lot closer to it than I was before my mathematical studies.) Since mathematics opened before my eyes and mind the most beautiful world I have ever known (except music, maybe), I am not so very sad about me losing this ability.
When I was a child, I was a synesthete. 1 was black for me, 2 was yellow, three was red ... I still remember, but I have lost the ability itself. During my childhood, someone could have said "four", and I would immediately have seen a green four inside my head. It worked with letters. It worked with words.
At that time, I thought that was perfectly normal. I thought everybody saw these colors. I didn't know it is a special ability, nor did I know it is a medical term (not in the negative sense). If I had, I would have protected this gift. Now I have lost it. That I am sad about, because I could have done such wonderful things with it, especially since I have become an artist.
But I digress, as I always do. The question was: What is my favourite number. I have thought about that, and two numbers whose incredible or at the very least interesting properties I have discovered in the course of my mathematical research have come to my mind:
2
What is so special about two? A lot. Check out this Wikipedia article if you want to know everything. (Although I doubt that every special property of 2 can be found on Wikipedia.) But what never ceases too fill me with delight is the fact that
2 is the only number for which this is true. I guess that readers will understand the first three terms, but what about the fourth? Well, as you know, the basic operator in algebra is addition. Multiplication is an extension of addition: a * b simply means that a is added (b - 1) times to itself. It's the same with exponentation: a ^ b is a multiplied (b - 1) times by itself.
It didn't take mathematicians long to figure out that one can continue this game forever; let's define an operator a ^^ b, which simply means that the operation ^ a is applied (b - 1) times to a, etc.:
a * b means: The operation + a is applied (b - 1) times to a.
a ^ b means: The operation * a is applied (b - 1) times to a.
a ^^ b means: The operation ^ a is applied (b - 1) times to a.
a ^^^ b means: The operation ^^ a is applied (b - 1) times to a.
...
This is exactly what the arrow notation means: Our symbol ^ is simply replaced with an arrow, whereas the n indicates the number of arrows. Therefore, the equation 2 + 2 = 2 * 2 = 2 ^ 2 = 2 ^^ 2 = ... is true. Pretty amazing, come to think of it. There is no natural number except 2 with this property.
(By the way: These non-standard operators are called hyperoperators. German mathematician Wilhelm Ackermann used hyperoperators in a famous and truly amazing function named after him. Read about it here. Maybe I'll write a blog entry on it sometime.)
It didn't take mathematicians long to figure out that one can continue this game forever; let's define an operator a ^^ b, which simply means that the operation ^ a is applied (b - 1) times to a, etc.:
a * b means: The operation + a is applied (b - 1) times to a.
a ^ b means: The operation * a is applied (b - 1) times to a.
a ^^ b means: The operation ^ a is applied (b - 1) times to a.
a ^^^ b means: The operation ^^ a is applied (b - 1) times to a.
...
This is exactly what the arrow notation means: Our symbol ^ is simply replaced with an arrow, whereas the n indicates the number of arrows. Therefore, the equation 2 + 2 = 2 * 2 = 2 ^ 2 = 2 ^^ 2 = ... is true. Pretty amazing, come to think of it. There is no natural number except 2 with this property.
(By the way: These non-standard operators are called hyperoperators. German mathematician Wilhelm Ackermann used hyperoperators in a famous and truly amazing function named after him. Read about it here. Maybe I'll write a blog entry on it sometime.)
144
I like the 144 because it is one out of only three numbers which are both Fibonacci numbers and square numbers. The other two are 0 and 1, and they are kind of boring in context with this property because it is so obvious for them. Fibonacci numbers are a quite well-known concept, as are square numbers. I'll just briefly explain them; square numbers are simply the sequence zero squared, one squared, two squared, etc.: 0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, ...
Fibonacci numbers are generated like this:
1. The first two Fibonacci numbers are 0 and then 1.
2. The next Fibonacci number is generated from the predecessing Fibonacci number by adding it to its own Fibonacci predecessor.
The generated sequence is 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, ...
Fibonacci numbers are amazingly often prime numbers (numbers divisible only by one and the number itself):
0, 1, 2 is prime, 3 is prime, 5 is prime, 8, 13 is prime, 21, 34, 55, 89 is prime, ...
(By the way, it is one of those famous unsolved problems in mathematics if there are infinitely many Fibonacci primes. If you have the time and want your name to become immortal, go for it.)
Because there are so many Fibonacci primes, it is very improbable that there is a large quantity of "Fibonacci squares". This was probably the first question I investigated in a serious mathematical way. It took me some time, and in the end I just used a formula known for almost 300 years, but it kept me interested and made me go deeper into the subject. And I am very, very glad I did that. Mathematics is a world of infinite beauty.
Fibonacci numbers are generated like this:
1. The first two Fibonacci numbers are 0 and then 1.
2. The next Fibonacci number is generated from the predecessing Fibonacci number by adding it to its own Fibonacci predecessor.
The generated sequence is 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, ...
Fibonacci numbers are amazingly often prime numbers (numbers divisible only by one and the number itself):
0, 1, 2 is prime, 3 is prime, 5 is prime, 8, 13 is prime, 21, 34, 55, 89 is prime, ...
(By the way, it is one of those famous unsolved problems in mathematics if there are infinitely many Fibonacci primes. If you have the time and want your name to become immortal, go for it.)
Because there are so many Fibonacci primes, it is very improbable that there is a large quantity of "Fibonacci squares". This was probably the first question I investigated in a serious mathematical way. It took me some time, and in the end I just used a formula known for almost 300 years, but it kept me interested and made me go deeper into the subject. And I am very, very glad I did that. Mathematics is a world of infinite beauty.
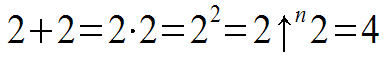

 RSS Feed
RSS Feed